Fluid dynamics analysis for a production line

Nestor Antonio  Flores Martínez; Valentín Guzmán  Ramos; Ricardo Chapa  García

doi:10.59400/mea.v2i1.438

Fluid dynamics analysis for a production line

Nestor Antonio Flores Martínez Facultad de Ciencias Físico-Matemáticas, Universidad Autónoma de Nuevo León, San Nicolás de los Garza 66455, Nuevo León, Mexico
Valentín Guzmán Ramos Facultad de Ciencias Físico-Matemáticas, Universidad Autónoma de Nuevo León, San Nicolás de los Garza 66455, Nuevo León, Mexico
Ricardo Chapa García Universidad Tecnológica General Mariano Escobedo, Escobedo 66050, Nuevo León, Mexico

Ariticle ID: 438

1274 Views, 77 PDF Downloads

DOI: https://doi.org/10.59400/mea.v2i1.438

Keywords: magnetic filters; turbulence; mass flux; fluid dynamics; industry; production line; fluid pressure

Abstract

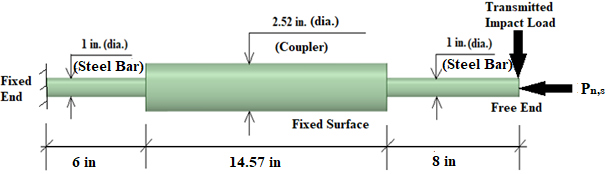
Magnetic filters are used on companies for mass flux and volume flux values that help to cover day-to-day production. This work begins by describing the problem of magnetic filter fractures as of industrial interest. A brief description of how magnetic filters must work on applications according to requirements on the process line. On the next section, it is possible to find the explanation of structural fractures and mistakes on the design or data sheet, which are usually not considered in guaranteed policy. Are mentioned more than one math modeling, taking the simplest to show the effective value of maximum pressure tolerated on magnetic filters differs from estimated for controlled conditions. Solutions shown in the article are a first approach to a future general model theory that could explain how applied science works in a factory where there is no time to stop production. This work relates the math model and some values to application, meaning both the project hypothesis modeling and factories requirements for their equipment. Results depict volume, velocity, density, and pipeline relation to the pressure increase over the values on the data sheet from quality department tests. Conclusions describe the connection of the math model analysis with real situations on the application or possible structural damage. And its relationship with the proper function of magnetic filters for the purpose of lifespan analysis on future works. This work is a proof that in a few weeks, companies can have a solution for their problems, letting them buy some time to avoid any future issues.

References

[1] Spevakova I, Ruditser L, Eroshenko V, et al. New Magnetic Filter for Chemical Industry. Magnetic and Electrical Separation. 1993; 5(1): 17-32. doi: 10.1155/1993/82842

[2] Szatyłowicz E, Skoczko I. Magnetic Field Usage Supported Filtration Through Different Filter Materials. Water. 2019; 11(8): 1584. doi: 10.3390/w11081584

[3] Flow Ezy Filters. Magnetic filtration: Sub-micron filtration for industrial fluids in precision applications. Available online: https://www.flowezyfilters.com/pdfs/magnetic/FlowEzyMagneticFiltration_master_catalog.pdf (accessed on 10 August 2023).

[4] Eclipse Magnetics. Magnetic filtration systems: Sub-micron filtration for industrial fluids in precision machining & wash system applications. Available online: https://www.eclipsemagnetics.com/site/assets/files/23188/filt_-_etna_v14_english_2020.pdf (accessed on 10 August 2024).

[5] Endoh S, Yamaguchi K. Characteristics of pressure drop across spongy nickel filter in magnetic separation process. Powder Technology. 1985; 45: 35-41.

[6] Alton Everest F. The Master Handbook of Acoustics, 4th ed. McGraw Hill; 2000. pp. 50-64,110-115.

[7] Aris R. Equations of Motion and Energy in Cartesian Coordinates. In: Vector, Tensors, and the Basic Equations of Fluid Mechanics. Dover Publications; 1989.

[8] Tam AC. Applications of photoacoustic sensing techniques. Reviews of Modern Physics. 1986; 58(2): 381-431. doi: 10.1103/revmodphys.58.381

[9] Bhattacharya K, Banerjee S, Mondal NK. Analytical computation of process noise matrix in Kalman filter for fitting curved tracks in magnetic field within dense, thick scatterers. The European Physical Journal C. 2016; 76(7). doi: 10.1140/epjc/s10052-016-4243-1

[10] Morfey CL, Sorokin SV, Gabard G. The effects of viscosity on sound radiation near solid surfaces. Journal of Fluid Mechanics. 2011; 690: 441-460. doi: 10.1017/jfm.2011.449

[11] Rosenhouse G. The Essence of Noise in Natura with Reference to Acoustics. WIT Transactions on Ecology and Environment; 2012. p. 160.

[12] Rhoads BL. River Dynamics: Geomorphology to Support Management, 1st ed. Cambridge University Press; 2020. pp. 75-95.

[13] Arteaga Marín J, Benavidez Muñoz H. Velocity profiles in a free-surface flow system with a hydraulic rotational vortex (Spanish). ESPAMCIENCIA. 2018; 9(1): 33-42.

[14] Newland DE. Introduction to probability distributions and averages. In: An Introduction to Random Vibrations, Spectral and Wavelet Analysis, 3rd ed. Dover Publications; 2005.

[15] Newland DE. Joint probability distributions, ensemble averages. In: An Introduction to Random Vibrations, Spectral and Wavelet Analysis, 3rd ed. Dover Publications; 2005.

[16] Newland DE. Correlation. In: An Introduction to Random Vibrations, Spectral and Wavelet Analysis, 3rd ed. Dover Publications; 2005.

[17] Newland DE. Spectral density. In: An Introduction to Random Vibrations, Spectral and Wavelet Analysis, 3rd ed. Dover Publications; 2005.

Published

2024-02-15

How to Cite

Flores Martínez, N. A., Ramos, V. G., & García, R. C. (2024). Fluid dynamics analysis for a production line. Mechanical Engineering Advances, 2(1), 438. https://doi.org/10.59400/mea.v2i1.438

Download Citation

Issue

Vol. 2 No. 1 (2024)

Section

Article

This work is licensed under a Creative Commons Attribution 4.0 International License.

In the paper, the MATLAB-Simulink model of simulation of operation of the fast light innovative regional train from “Serbian Railways” in traction and braking mode is exposed where changes are observed: stator currents of three-phase traction motors, traction electric motor speeds and electric multiple units, electromagnetic torque on the rotor shaft of the traction electric motor, and direct current bus voltage. The model allowed review of the listed parameters for: different allowed values of contact network voltage and total voltage distortion at the place of connection of the electric multiple unit to the contact network; different mechanical loads of the electric multiple unit and traction electric motor; and different train speeds and rotations of traction electric motors. Appropriate conclusions were made through the analysis of the simulation results obtained.

This paper defines vehicles and buildings as main sources of United Kingdom (UK) carbon dioxide (CO₂) and seeks to cut such emissions using green hydrogen made from combined wind and solar energy. Combustion vehicles powered by fossil petroleum emit near half of UK climate-warming CO₂ while buildings heated by natural gas provide a third. First, current UK grid problems are defined: Electricity, gas and petroleum grids. Refueling green vehicles has been a particular problem. Then experiments on the private wire community of Keele University show how green hydrogen could integrate both green vehicles and buildings. Next, the model supply chain is planned and tested. Finally, experiments and calculations are outlined, analyzing the optimum system design criteria proposed. We conclude that economic green hydrogen can displace petroleum in vehicles, while powering buildings instead of natural gas. Also, the prospect in 2024 is that profits can be made all along the green hydrogen supply chain, such that new businesses involved in local private clean communities can cost less than the National Grid monopoly and other dominant fossil energy companies.

Presently, dynamic impacts occurrences caused by car crashes have been frequently reported. Statistical results from different articles indicate that vehicle crashworthiness of bridge pier supersedes the other events. However, majority of the published articles focusses on sustainability and finding severity of distressed pier due to impact correlating faster construction methods, like accelerated bridge construction (ABC). This article is an attempt to examine post impacted behavior of a commonly used connector in ABC for resisting short duration shock. Static and dynamic performance analyses of a connector embedded within a coupler system has been examined. A representative ABC pier with the standardized and selected material properties collected from manufacturer’s data has been utilized. Coupler composite materials consisting of a hollow uniform cross-section cast iron cylinder filled up with specified concrete grout conforms higher strength. The performance examination has been conducted by numerical modeling using finite element method (FEM). A commercially used software ANSYS has been utilized for carrying out the simulations. To investigate the post impact dynamic behavior, mesh-independent studies seems inevitable and hence are executed to evaluate dynamic impact factor (DIF). Sensitivity studies are carried out for validating results in precision. The DIF of the main reinforcing tensile steel embedded into the grout placed inside the connector has been determined. Results captured from simulations to identify material properties at plastic stage in sustaining such load have been conducted. The results provide significant information that aids opting for selecting suitable connectors for attaining help the design offices.